Wire Maze Electricity DIY STEM Kit
$9.99$5.55
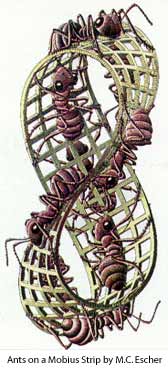 Very roughly a surface is orientable if it has two sides so that, for example, is
it possible to paint it with two different colours. A sheet of paper or the
surface of a sphere are examples of orientable surfaces. A Mobius strip is a
non-orientable surface: you can build one with a strip of paper (twist the strip
and glue end together to form a ring) and verify that it has only one side: it is
not possible to paint it with two colours.
Very roughly a surface is orientable if it has two sides so that, for example, is
it possible to paint it with two different colours. A sheet of paper or the
surface of a sphere are examples of orientable surfaces. A Mobius strip is a
non-orientable surface: you can build one with a strip of paper (twist the strip
and glue end together to form a ring) and verify that it has only one side: it is
not possible to paint it with two colours.

 'The greatest good will come from the technical improvements tending to unification and harmony.'
'The greatest good will come from the technical improvements tending to unification and harmony.'